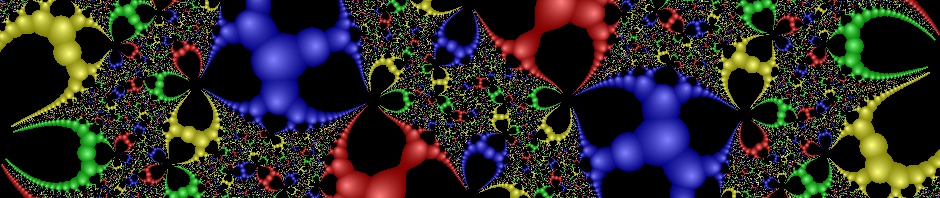
This image illustrates the behavior of a particular quantum circuit. To be precise, this circuit is an iterated quantum controlled NOT gate (QCNOT) with a twist of 45.5 degrees in the Hopf fiber containing the identity. This means that one of the input qubits, which may be taken to be the control qubit for definiteness, is subjected to a particular unitary transformation before being fed into QCNOT.
should really be thought of as an element of the projective special unitary group
but we can be slightly redundant and view it as an element of
If we identify
with the three-dimensional sphere
then we may use the Hopf fibration
to twist (i.e., rotate) a given transformation in its Hopf fiber, which is a circle. In this case,
is given by twisting the identity matrix
by
degrees in its Hopf fiber.
The image was created using the software Fractal Domains. It shows a portion of the complex plane, viewed as a coordinate chart on the Riemann-Bloch sphere, which may be identified with the space of single-qubit states. The center of the chart is at the origin, and the top right corner is at roughly For each single-qubit state
, the pair
is fed into the QCNOT gate and the procedure is iterated using the target output qubit. Note that although we cannot create a single device to duplicate every possible qubit state
because of the no-cloning theorem, we can still choose any particular state we like and create as many copies as desired. The colors represent different orbits of a representative region of the plane. The chaotic behavior arises from the nonlinearity of the composite operation